how to find the slope
How do we find the slope of a linear equation? Let's dive in and discover!

While mathematics is often grouped with the sciences, in some respects, math is a beautiful language. For instance, when comparing how something grows, one might say it grows slowly, moderately, or quickly. But, how slowly? How quickly? Using mathematics, we can describe the growth of functions using slope.
In this review, we will explain how to find slope, focusing on linear functions. By the end, you will know how to find the slope of a line. This includes how to find slope with two points, how to find slope using a graph, and how to find the slope of an equation.
What is slope?
Slope describes the rate of change or "steepness" of a line.
A linear function will look like a straight line when graphed. Here are some examples of linear equations and their slopes (click images to expand):
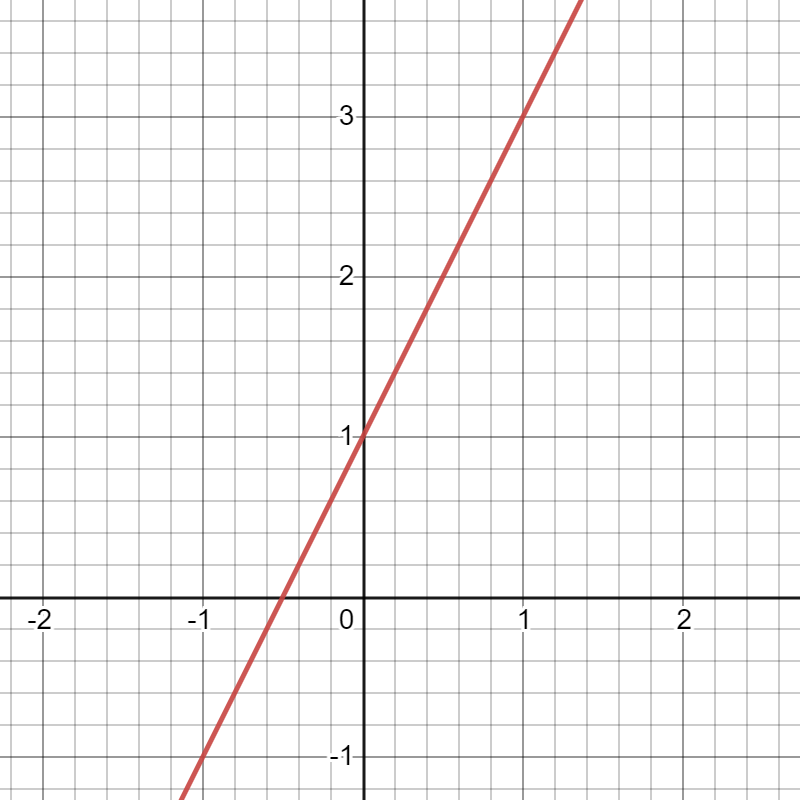
Slope: 2

Slope: 7
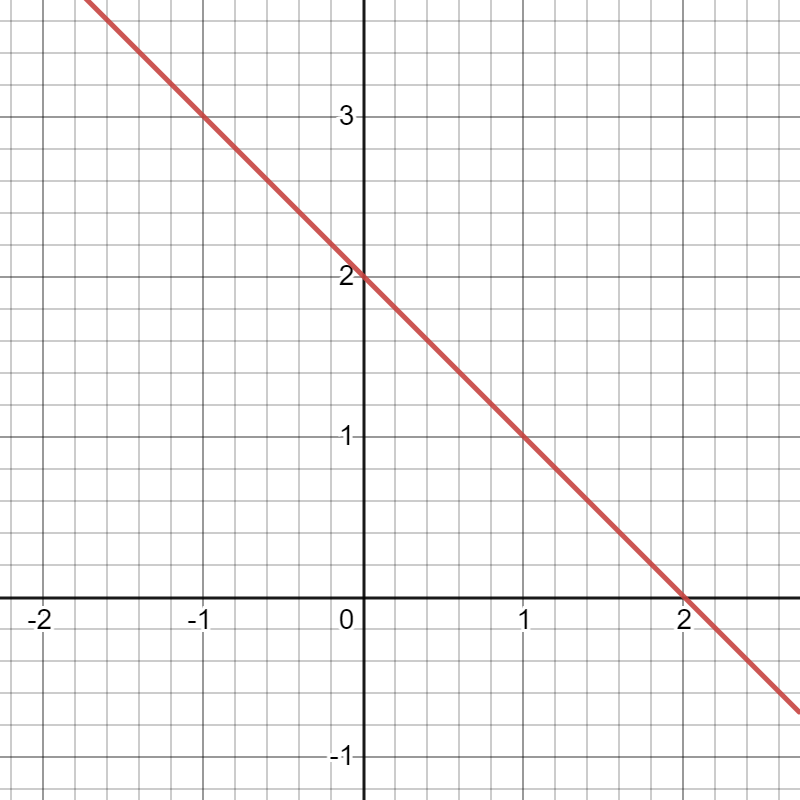
Slope: -1
What is the formula for slope?
For two points with the coordinate (x_1, y_1) and (x_2, y_2), the formula for slope is:
m=\dfrac{y_2-y_1}{x_2-x_1}
"Rise Over Run"
The slope of a line can be described as rise over run .
The rise is the vertical change in y and the run is the horizontal change in x.
In the slope formula above, the letter m represents the slope.
Below is a visual to understand what we mean by "rise" (vertical change shown in the dotted blue line) and "run" (horizontal change shown in the dotted green line):

Negative vs Positive Slope of Linear Equations
Positive Slope
When a function has a positive slope, the line is moving up from left to right. In other words, as the value of x increases, the value of y also increases. The greater the slope of a positive-slope line, the steeper the line is.
For example, a slope of 7 would create a steeper line than a slope of 2.
Here are some examples of lines with positive slopes (click images to expand):
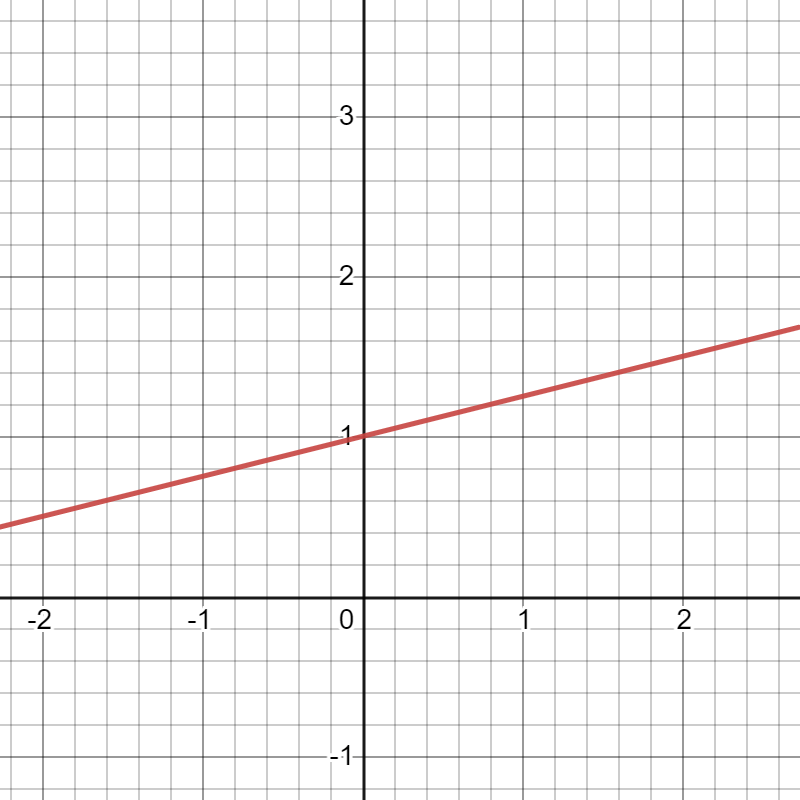
Slope: \frac{ 1}{4 }
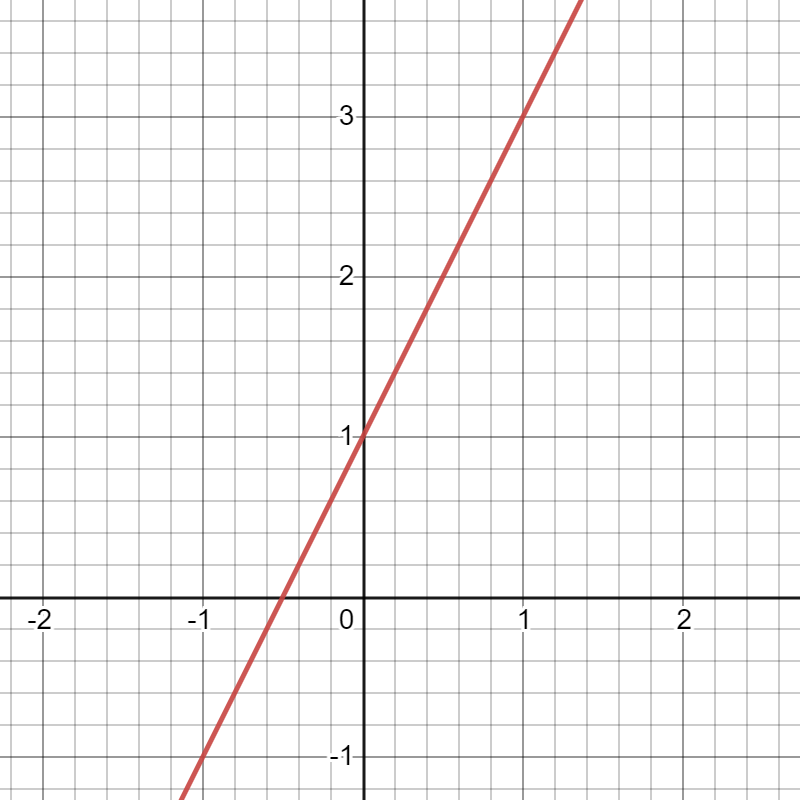
Slope: 2

Slope: 7
Negative Slope
When a function has a negative slope, the line is moving down from left to right. In other words, as the value of x increases, the value of y decreases. The lower the slope of a negative-slope line, the steeper the line is.
For example, a slope of -10 would create a steeper line than a slope of -2.
Here are some examples of lines with negative slopes (click images to expand):
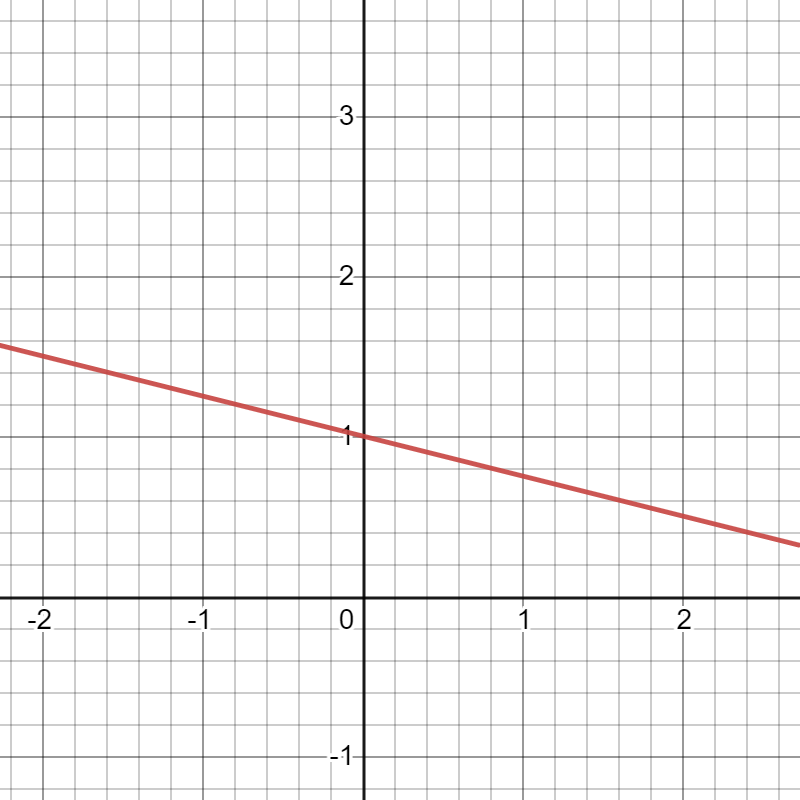
Slope: - \frac{ 1}{4 }
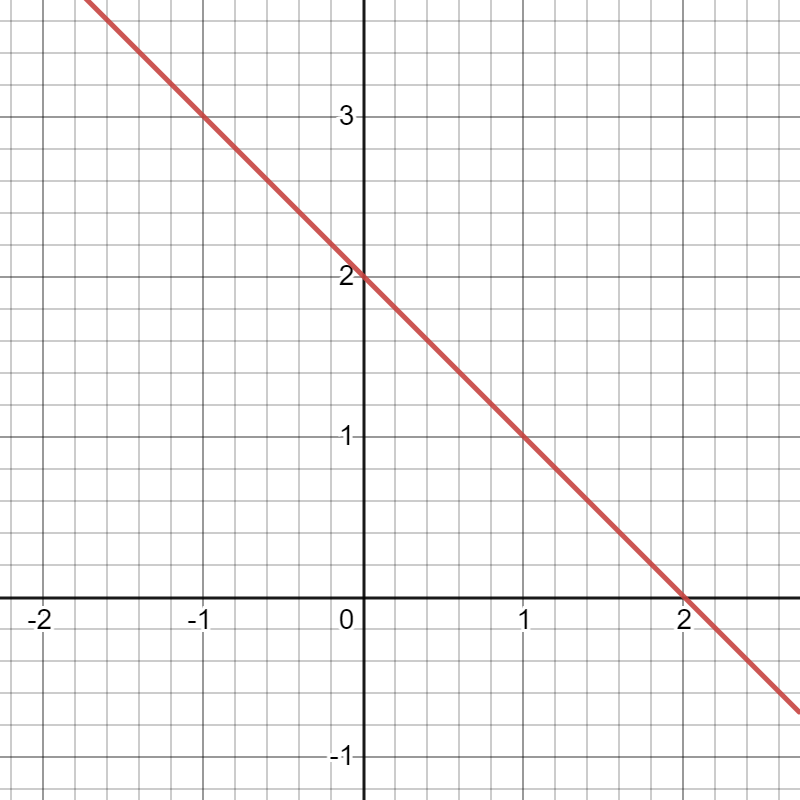
Slope: -1
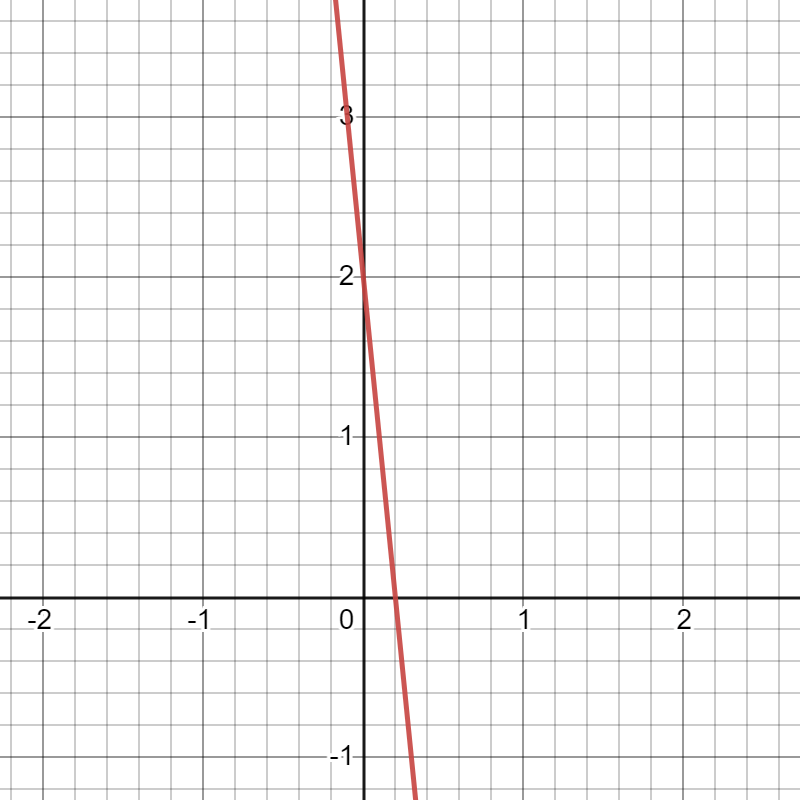
Slope: -10
Find Slope from Two Points (Example)
We will now talk about how to find slope with two points.
Let's begin with a simple example. We will use Point A, (5,8) and Point B (1,4) .
Remember, we can start with the formula for slope:
m=\dfrac{y_2-y_1}{x_2-x_1}
Before we can substitute the values into the equation, we should label the points.


Now, we can substitute 5 for x_1, 8 for y_1, 1 for x_2 and 4 for y_2.
\dfrac{y_2-y_1}{x_2-x_1}
\dfrac{4-8}{1-5}
And next, we simplify to find the slope of the line.
\dfrac{-4}{-4} = 1
Therefore, the slope of a line going through Point A, (5,8) and Point B (1,4) would be 1. That was an example of how to find the slope when given two points.
If helpful, here's a quick video explaining how to calculate the slope when given two points:
Find Slope From a Graph (Example)
To calculate the slope of a line from a graph, we use the graph to determine the rise (the vertical change) and the run (the horizontal change)
To begin, we select two points on the graph that are easy to identify. We can consider the line y=2x-1 as an example.
On the graph shown below, the red line represents y=2x-1. We can identify the points (1,1) and (2,3) on this line.
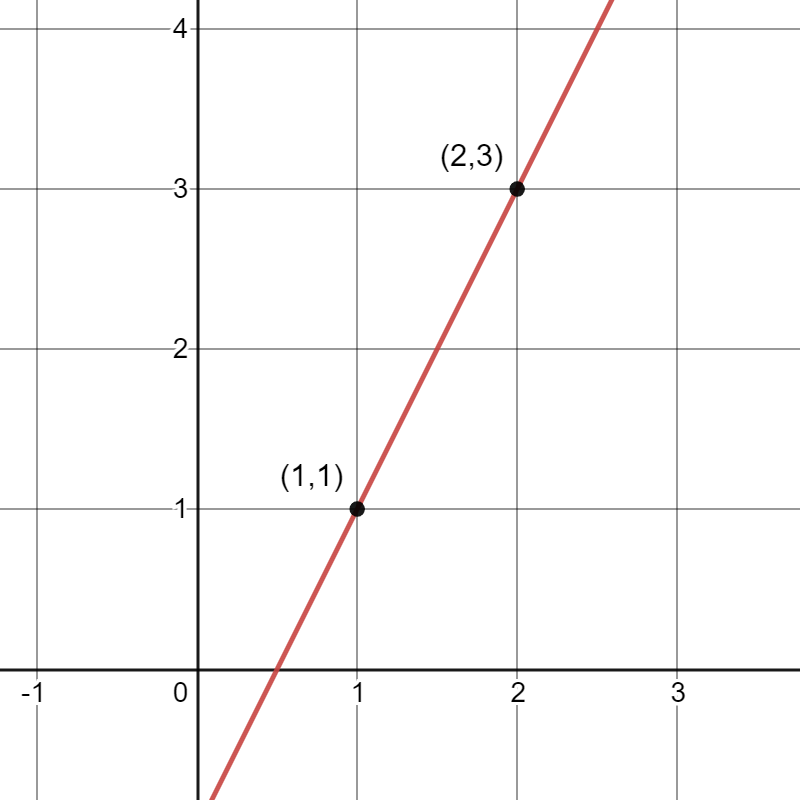
Now, we can count the vertical distance between the points to determine the rise. In the following image, the vertical distance of {2} is in blue, shown below:
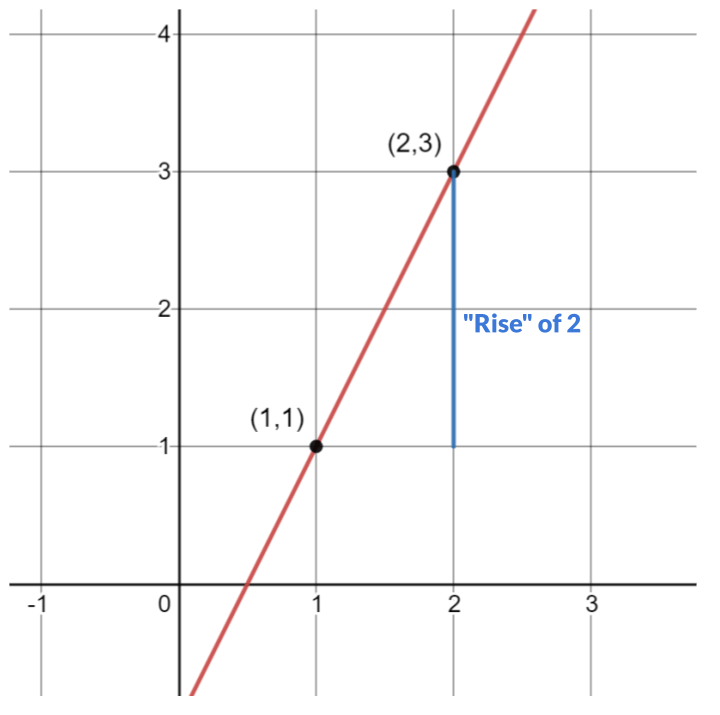
We also must count the horizontal distance between the points to determine the run. In the following image, the horizontal distance of {1} is in green.
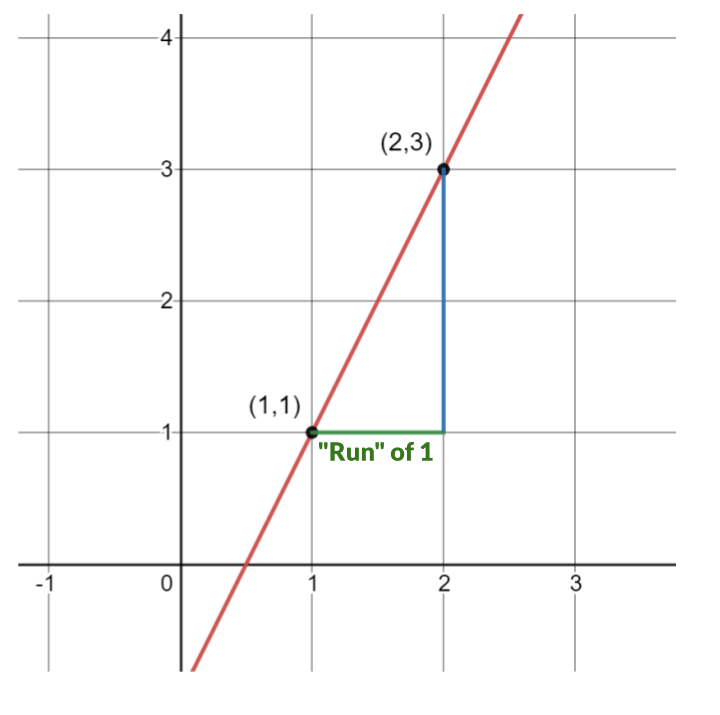
Therefore, the rise is {2} and the run is {1} . The slope is rise over run or \dfrac{2}{1} which simplifies to 2.
We now know how to find slope using a graph!
For those who prefer videos, here's a quick clip showing this method of finding slope from a graph:
Find Slope From an Equation (Example)
If you want to know how to find the slope of a linear equation, you must first know that linear equations are often written in slope-intercept form.
In slope-intercept form, an equation is written:
y=mx+b
The letter m represents the slope and the letter b represents the y-intercept.
To determine how to find slope, you must identify the coefficient in front of x.
For example, consider the equation:
y=7x+10
The coefficient in front of x is 7. Therefore, we know the slope of the line y=7x+10 is 7.
If you encounter an equation in a different form, simply solve the equation for y and write the equation in slope-intercept form. Then, you can use this method to determine the slope.
In addition, this article has some great real-life applications of slope.
And here's a quick video on how to find the slope of a line when given an equation:
Slope of Horizontal and Vertical Lines
When learning how to calculate slope, there are two unusual cases that require some special attention: horizontal and vertical lines.
Horizontal Lines
If you are finding slope from two points and you end up with zero in the numerator, you have found the slope of a horizontal line.
This slope of a horizontal line is always 0. The equation of a horizontal line is y=a where a is a real number. Here are two examples of horizontal lines shown in red (click images to expand):
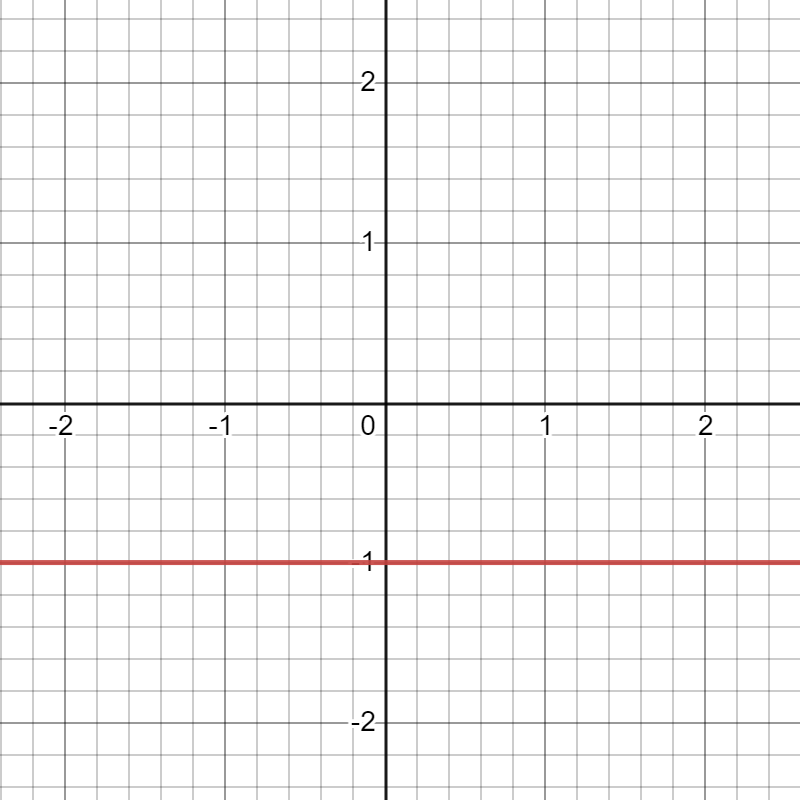
Slope: 0
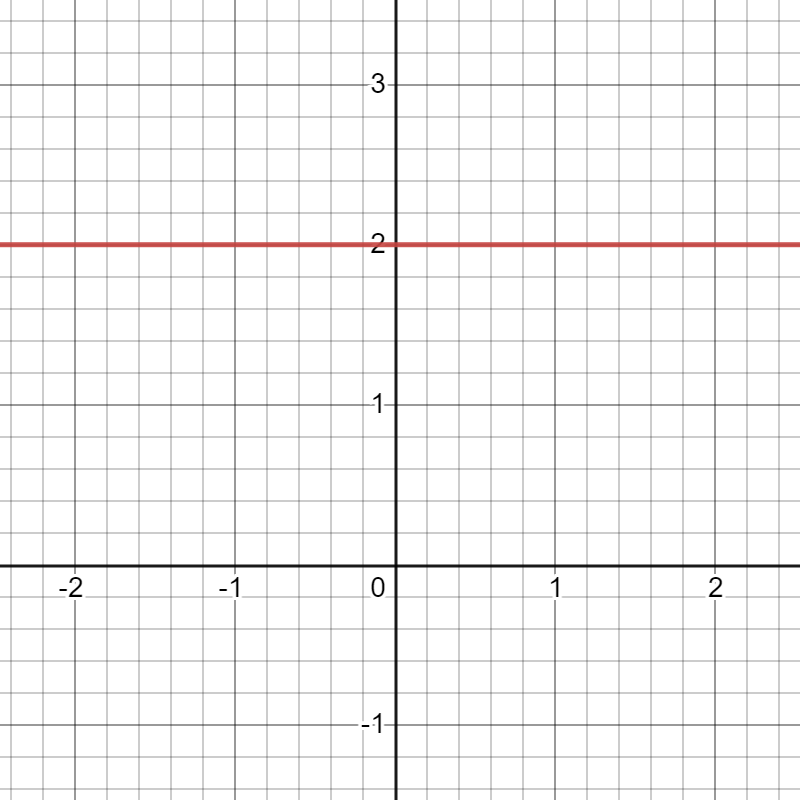
Slope: 0
Vertical Lines
If you are finding slope from two points and you end up with zero in the denominator, you have found the slope of a vertical line.
This slope of a vertical line is always undefined.
The equation of a vertical line is x=a where a is a real number. Here are two examples of vertical lines shown in red (click images to expand):
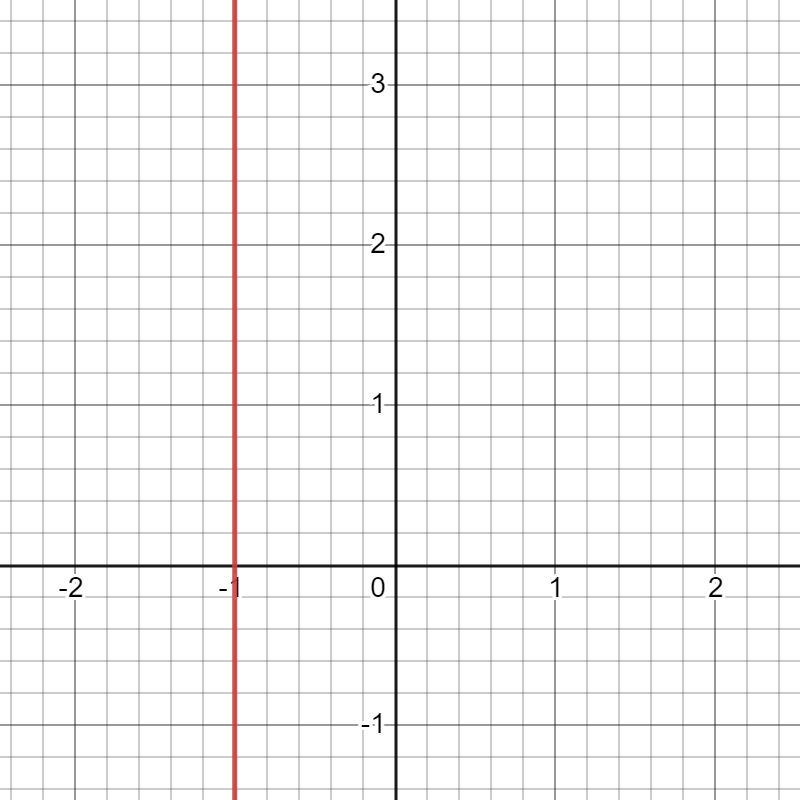
Slope: undefined
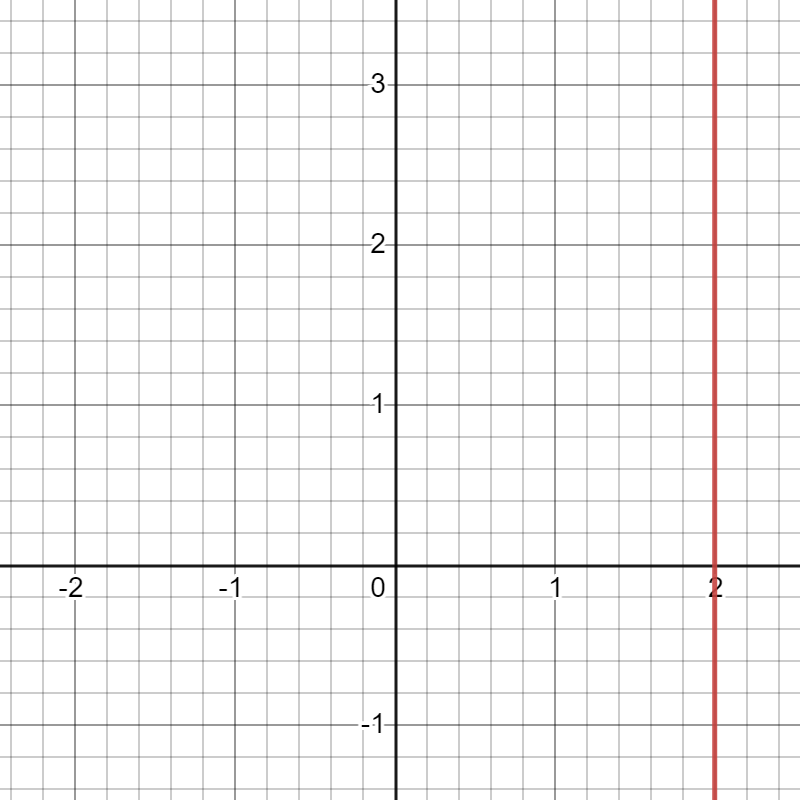
Slope: undefined
Summary: Slope of Linear Equations
- Understanding how to find slope can help explain the world around us!
- The slope of a linear equation is the "rise over run", or the change in y over the change in x.
- The equation to find the slope of a line is:
m=\dfrac{y_2-y_1}{x_2-x_1}
- Horizontal lines always have a slope of zero and vertical lines always have an undefined slope.
Free Practice Problems With Slope
Now that we know how to find slope with two points, how to find slope using a graph, and how to find the slope of an equation, it's time to practice our new skills.
In addition, you can explore more of our free detailed review guides of important Algebra 1 topics.
how to find the slope
Source: https://www.albert.io/blog/how-to-find-slope/
Posted by: michiethadell77.blogspot.com

0 Response to "how to find the slope"
Post a Comment